문제

• https://school.programmers.co.kr/learn/courses/30/lessons/12978

코드

function solution(N, road, K) {
  const arr = Array(N + 1).fill(Number.MAX_SAFE_INTEGER);
  const lines = Array.from(Array(N + 1), () => []);

  road.forEach((value) => {
    // 연결되어 있는 경로를 모두 lines배열에 저장한다.
    let [a, b, c] = value;
    lines[a].push({ to: b, cost: c });
    lines[b].push({ to: a, cost: c });
  });

  let queue = [{ to: 1, cost: 0 }];
  arr[1] = 0;

  while (queue.length) {
    let { to } = queue.pop();

    lines[to].forEach((next) => {
      // 모든 경로를 탐색
      if (arr[next.to] > arr[to] + next.cost) {
        // 기존에 경로의 값보다 우회하는 값이 더 작으면 해당 값을 저장함
        arr[next.to] = arr[to] + next.cost;
        queue.push(next);
      }
    });
  }

  return arr.filter((item) => item <= K).length; // 경로의 제한인 K보다 cost가 작은 경로의 수를 반환을 함
}

// 객체 버전
function solution(N, road, K) {
    const ans = Array(N).fill(Number.MAX_SAFE_INTEGER);
    const routes = {};
    
    for(let route of road) {
        const [v1, v2, time] = route;
        if(routes[v1]) routes[v1].push([v2, time]);
        else routes[v1] = [[v2, time]]
        if(routes[v2]) routes[v2].push([v1, time]);
        else routes[v2] = [[v1, time]];
    }
    
    const queue = [[1, 0]];
    ans[0] = 0;
    while(queue.length) {
        const cur = queue.pop()[0];
                
        routes[cur].forEach(route => {
            const [next, time] = route;
            
            if(ans[cur-1] + time < ans[next-1]) {
                ans[next-1] = ans[cur-1] + time;
                queue.push([next, ans[next-1]]);
            }
        })
    }
    
    return ans.filter(x => x <= K).length;
}

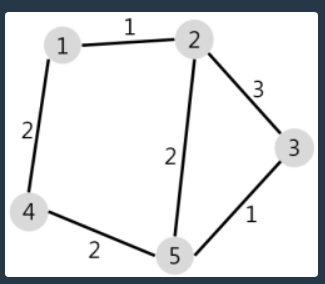
다익스트라 알고리즘

• 주로 최단거리를 구할 때 사용한다. (네비게이션 등)
• 이런 그림 나왔다 하면 다익스트라 생각하면 된다.
  

ans 배열 초기화

• ans를 미친 무한 수로 N의 개수만큼 저장
• 나중에 바꿔 줄 예정이다.

queue를 이용한 풀이

• 처음에 DFS를 이용해 풀려고 했는데 시간초과 개떡같이 나서 실패한 문제
• 먼저 이어져있는 길과 시간을 배열에 담는다. (난 객체가 편해서 객체로 함)
• queue에 시작점인 1과 초기시간인 0을 넣어준다.
• 1번은 시간이 0이니까 ans[0] 혹은 ans[1]을 바꿔준다.(밑에 내가 푼 풀이가 직관성은 떨어지는데 난 더 좋다. 암튼 내 자식이 더 좋음)
• 다음 이어질 곳의 시간과 total이 ans의 next 위치보다 작으면 바꿔주고, queue에 푸시해준다.

filter로 마무리

• K를 언제 사용하나 했는데 마지막에 filter로 걸러준다.
• 처음에 풀 땐 ans에 넣을 때마다 검증했는데 위 방법이 더 낫다. 왜냐면 내 방법은 시간초과로 검증도 못해봤다.